Acoustic modelling is generally used in auralisation to predict sound propagation behaviour in a space that does not as yet exist, and so is a key design process in the development of new performing arts venues, where acoustic quality is critical (Schroeder 1973). It is also used to predict the acoustic consequences for refurbishments planned in existing venues. Traditionally, the model might in fact be a reduced-scale construction of the actual building, complete with miniature loudspeakers (for sound sources) and microphones (for the listener's ears), with the audio signals scaled up in frequency accordingly to compensate for the change in physical dimensions (Polack et al. 1993). Such techniques are now rarely used owing to the high level of skill needed in the construction, not to mention the time required to build them, the high costs involved and the limitations of the miniature audio systems used.
Computer-based acoustic modelling (Välimäki et al. 2012; Savioja and Svensson 2015), is therefore much more established, based on 3D computer-aided design techniques, and makes it possible to use a computer-generated visualisation and from this derive an auralisation. Despite the flexibility that this implies (it is much easier to edit, change and experiment with the design of a computer-based environment than with a comparable scale model, or even the real thing), the accuracy of the result is still only as good as the mathematical techniques that are used to describe how sound behaves within this virtual 3D space. As yet there is no perfect solution to this problem. Most existing commercial software makes use of one or more geometric acoustic modelling techniques (Savioja and Svensson 2015). Here, sound is assumed to travel in straight lines, similar to a ray of light, and sound paths are calculated from source to listener based on how these predicted paths interact with the surrounding geometry of the environment and reflect from walls and objects (Figure 2). The result is a close approximation to the impulse response of the modelled environment for a given set of conditions, although results at low frequencies are often less accurate, as geometric acoustic methods are less able to model the wave-like behaviour of sound at these frequencies. This problem is an area of active research and an alternative approach is to use a numerical method to solve the underlying equations of wave motion (van Mourik and Murphy 2014). Although more accurate, such methods are too expensive computationally to offer a complete solution, taking hours, days, or weeks to arrive at the final result, and hence hybrid methods, taking advantage of both approaches, are also an area of current research (Southern et al. 2013).

Despite there being limitations to the methods used in acoustic modelling, it is still possible to get very close to an optimal result, and certainly to a point that the resulting auralisations are considered to be perceptually plausible, or 'good enough'.
Acoustic measurement for auralisation is the real-world equivalent of acoustic modelling. As with computer-based modelling, the goal is to obtain a set of acoustic impulse responses from the measured space that can be used for further analysis, to better understand how the space has an impact on sounds heard within it, or for auralisation. Although it is possible to arrive at an approximation of the acoustic impulse response by using a balloon pop or starter pistol as the sound source excitation, recorded at the required listener position, it is much more common to use an analytical signal played back through a loudspeaker. The method presented in Farina and Ayalon (2003), based on an exponential sine wave sweep through all frequencies of interest (typically 22Hz to 22kHz to cover the complete audio spectrum), is now widely used, with additional post-measurement processing applied to inverse filter the sweep signal to arrive at the required impulse response (Figures 3–5).


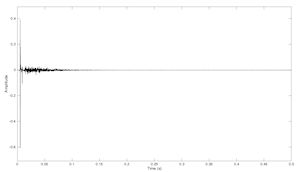
The loudspeaker used as the sound source is of some importance for an optimal result. Acoustic standards recommend an omnidirectional loudspeaker so that the measured space is excited equally in all directions (ISO3382-1 2009), although this is more usually applied for acoustic analysis rather than auralisation. Omnidirectional loudspeakers are often not ideal for auralisation – they have a non-flat frequency response that will colour the excitation signal and therefore also the recorded measurement, and at wavelengths (and hence higher frequencies) comparable or shorter than the loudspeaker driver diameter, actually become highly directional. Furthermore, auralisation is designed to simulate a specific sound played back in the measured space and the most commonly used acoustic signals (e.g. speech, musical instruments) have a particular directional characteristic. Hence an omnidirectional loudspeaker over-illuminates the environment with acoustic energy in a way that real acoustic sound sources rarely do. For this reason, high quality recording-studio monitor loudspeakers are often used (Farina and Ayalon 2003; Murphy 2005; 2006; Lopez et al. 2013) – they have a typically flat and extended frequency response with good coverage of both low bass and high treble ends, and a directional characteristic that is generally uniform with frequency. If a more omnidirectional excitation is required, it is possible to orientate the loudspeaker to different directions and sum across the results obtained (Shelley et al. 2013). Some equalisation of the recorded measurements might then be needed to account for too much bass energy in the resultant sum, as such loudspeakers tend to be more omnidirectional in this frequency range in any case (Figures 6–7).


A combination of microphones at the listener position to record the signal propagating through the space from the source loudspeaker makes it possible to capture a large amount of impulse response data in one pass. Getting access to a site for study is often difficult, and usually only a small window of opportunity is available away from normal activities, when both interior and exterior environments are quiet enough to enable high-quality acoustic measurements to be made. Hence it is important to gather as much data as possible in a short time frame. This approach was pioneered in Farina and Ayalon (2003), where a stereo microphone pair, a binaural dummy head microphone and an Ambisonic B-format Soundfield microphone are used together, in combination with a rotating turntable to automate the measurement process. This microphone array takes 36 sets of measurements over eight channels at 10-degree intervals. An alternative version was used in Murphy (2005; 2006), and forms the basis of most of the measurements available on OpenAIR. In this configuration a Soundfield microphone is positioned on a boom arm, 1m from the centre axis of the automated rotating turntable. A single Neumann KM140 cardioid microphone is situated with the capsule end 10.4cm from the centre axis, essentially one-half of an ORTF stereo microphone pair spaced 17cm apart at an angle of 110 degrees. Both microphones are set at a height of 1.5m and a rotation increment of 5 degrees is used (Figure 8). This simplifies the system used in Farina and Ayalon (2003), but still enables the 72 sets of five-channel impulse response information to be combined for a wide variety of surround-sound auralisation or acoustic analysis options.
As the OpenAIR database expanded to include outdoor environments, the measurement system used was simplified further for the sake of portability, and became based around a single Soundfield microphone (Figure 9). The Soundfield microphone used across all of these methods consists of a four-channel coincident array of microphone capsules that spatially samples (or records) the acoustic field at a given point. It is compact, simple and easy to use, does not require complex calibration, and gives flexible rendering options for decoding the impulse response measurements for many types of speaker configuration, including binaural sound via a further post-processing and signal transformation stage. Soundfield microphone recordings/measurements also form the basis of parametric spatial audio rendering techniques (Merimaa and Pulkki 2005; Berge and Barrett 2010) that have the potential to give better spatial accuracy for a wider group of listeners within a loudspeaker array, without having to resort to microphones based on more complex spatial arrangements and higher channel counts.


Internet Archaeology is an open access journal based in the Department of Archaeology, University of York. Except where otherwise noted, content from this work may be used under the terms of the Creative Commons Attribution 3.0 (CC BY) Unported licence, which permits unrestricted use, distribution, and reproduction in any medium, provided that attribution to the author(s), the title of the work, the Internet Archaeology journal and the relevant URL/DOI are given.
Terms and Conditions | Legal Statements | Privacy Policy | Cookies Policy | Citing Internet Archaeology
Internet Archaeology content is preserved for the long term with the Archaeology Data Service. Help sustain and support open access publication by donating to our Open Access Archaeology Fund.